What is Measure of Dispersion?

What is Measure of Dispersion?

Using different measures of central tendency, you can find out the mean value, but these measures do not explain the scattering of values near the mid value in a data series. The measures of dispersion can be used to study the dispersed values near the mean value.

Let us now discuss each measure of dispersion.

Range

Range represents the difference between the highest value and the lowest value in a data series. It is considered as a rough measure of variability because it depends on the size of the data series. When the highest (H) and/or the lowest (L) data point in a data series changes , the range also changes.

Coefficient Range = H – L / H + L

The formula used to calculate range is as follows:

Range = (Highest value of data series – Lowest value of data series)

Let us learn to calculate range with the help of the preceding example in which a group of 17 people rated a book on a 5-pointer scale, where 1 is the lowest rating and 5 is the highest rating. The rating given by the 17 people is as follows:

2, 5, 3, 4, 1, 5, 4, 3, 1, 2, 5, 4, 3, 2, 1, 5, 4

Now, you want to calculate the range for the data series.

To do so, you need to find the highest and lowest values of the data series. In the present case,

Highest value of data series = 5

Lowest value of data series = 1

Therefore, the range would be:

Range= (Highest value of data series – lowest value of data series)

Range = (5 – 1)

Range = 4

Therefore, the range of the ratings given by 17 people to a book is 4.

Mean Deviation

Mean deviation represents the extent of deviation of values from the mean. According to Clark and Schkade, average deviation is the average amount of scatter of the items in a distribution from either the mean or the median, ignoring the signs of the deviations. The average that is taken of the scatter is an arithmetic mean, which accounts for the fact that this measure is often called the mean deviation. Mean Deviation is used to measure variability across a data series.

The formula used to calculate Mean Deviation is as follows:

Mean Deviation (MD) = ∑|X_i – X|/n

Where X_i = Individual observation

X = Mean/Median/Mode

n = Number of observations

With the help of MD, you can also calculate the coefficient of MD. The coefficient of MD refers to the relative measure of dispersion that can be calculated by dividing MD with mean/median/mode.

The formula to calculate the coefficient of mean deviation is as follows:

Coefficient of MD = MD/X

Where M.D = Mean Deviation

X = Mean/Median/Mode

Let us understand the concept of MD and the coefficient of MD with the help of an earlier example in which you calculated the average weight of five friends. Table 8.2 shows the data used for calculating mean deviation:

People	Weight (kg)	|Xi – X|
Jenny	35	|35 – 38| = 3
People	Weight (kg)	|Xi – X|
Robert	40	|40 – 38| = 2
Ella	34	|34 – 38| = 4
Andy	39	|39 – 38| = 1
Eliza	42	|42 – 38| = 4
Total		14

X = 35 + 40 + 34 + 39 + 42 / 5 = 38

The formula to calculate MD is shown as follows:

Mean Deviation (M.D.) = ∑|X_i – X|/n

M.D. = 14/5

M.D. = 2.8

Coefficient of Mean Deviation = M.D./X

= 2.8/38
= 0.074

Therefore, the dispersion of the weight of five friends from the mean value is 2.8, Therefore, the weight of all friends is dispersed more or less by 2.8 kg from the average weight. The relative measure of weight is 0.074.

Standard Deviation

Standard Deviation is used to calculate the scattering of values in a given dataset. The symbol used to represent standard deviation is sigma (σ). Standard Deviation (SD) is the square root of variance of a data series. The formula used to calculate SD is as follows:

For research where entire population is considered,

SD of population σ = ( )

and σ = Parameter of the population

For research where only a sample is considered,

SD of Sample S = ( )

and S = Statistic of sample

Also not that the square of SD is called variance

Population variance = σ² and Sample variance = S

Sample statics is used to estimate population parameter. S² is an unbiased estimate of σ² .

If the observations are grouped into a frequency table, than the formula of SD and variance change as follow:

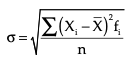
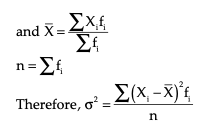
The coefficient of SD can be calculated by dividing SD with the mean of the series. It is a relative measure of dispersion. Let us understand the concepts of SD, the coefficient of SD, and the coefficient of variance with the help of an example. Suppose you want to calculate the standard deviation of the weight of five friends shown in the preceding example.